Precise tracking of star shows how the Milky Way’s central black hole curves space in its neighborhood
Einstein’s theory of general relativity and Newton’s theory of gravitation make slightly different predictions when it comes to objects orbiting a central mass. Now a consortium of researchers, led by the Max Planck Institute for Extraterrestrial Physics (MPE) and including astronomers from the Max Planck Institute for Astronomy (MPIA), has for the first time detected the so-called Schwarzschild precession, a specific general-relativistic effect that changes the orbit of a body around a central mass, for a star orbiting the supermassive black hole at the center of the Milky Way. The effect depends in part on the curvature of space around the central mass.

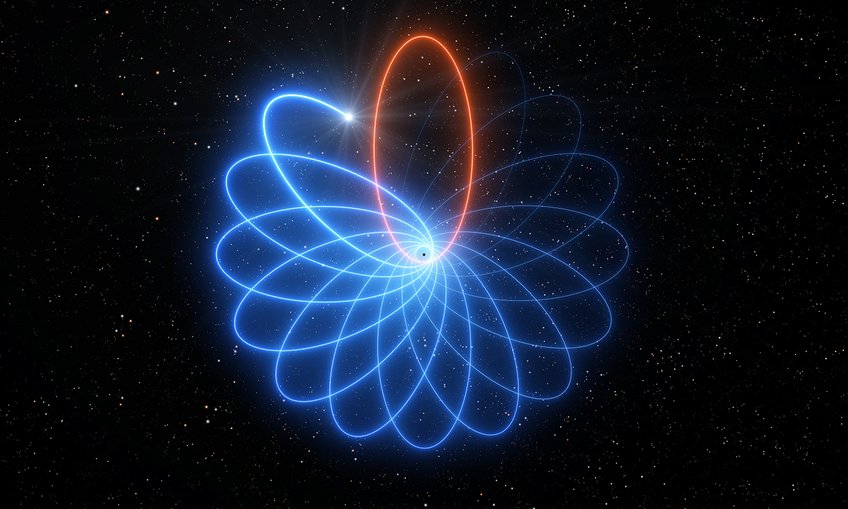
Artist's impression of the Schwarzschild precession of a stellar orbit around our galaxy's central black hole: the ellipse is transformed into a rosette, here shown in an exaggerated fashion.
Einstein's theory of general relativity, which links the properties of space and time with the actions of gravity, has just passed another test with flying colors: Its prediction of what should happen to a star's orbit as that star passes close to a black hole, in this case the supermassive central black hole of our Milky Way galaxy, have been demonstrated to be spot-on. The observations that were used to put general relativity to this new test are an impressive feat of observational astronomy in its own right: The measurements in question were only possible due to the GRAVITY instrument, installed at the Very Large Telescope (VLT) of the European Southern Observatory ESO. GRAVITY makes use of the wave properties of light in order to measure differences in stellar positions with an accuracy of up to 30 millionth of an arc second. With that accuracy, the instrument could distinguish two fireflies, 6 cm apart, sitting on the Moon. That accuracy has been crucial in detecting the general-relativistic effect, known as Schwarzschild precession, in the orbit of the star S2 around the galactic center.
Orbiting the central black hole
From our perspective, the central black hole of our home galaxy sits behind clouds of gas and dust, nearly 27000 light-years away. Because of the dust, it cannot be seen in visible light – only at longer wavelengths, which pass through the dust almost unhindered. Radio observations have long identified the black hole as the radio source “Sagittarius A*” (pronounced “Sagittarius A-star”), whose radio waves are produced by the black hole’s immediate surroundings of matter swirling around, and falling into, the black hole.
In the early 2000s, first infrared observations by a group led by Reinhard Genzel at the Max Planck Institute for Extraterrestrial Physics (MPE) showed the motion of stars close to the black hole, and thus the influence of the black hole’s strong gravity. Between then and now, researchers have been able to trace the orbits of more than a dozen stars, but there is one that is particularly well-studied: It is called “S2”, its observed orbit has been traced with great precision, and it passes particularly close to the black hole. In view of all this, S2 is an ideal test object for detecting relativistic effects of the central black hole on objects in its immediate vicinity.
The orbital period of an object orbiting a central mass depends on the object’s distance from the mass and on the value of the mass itself. Later observations by the Genzel group, and also by an independent group led by Andrea Ghez at UCLA, allowed the researchers to deduce from those stellar orbits the mass of the central black hole; the best modern value is around 4 million Solar masses.
Technological advances and new knowledge
Studies of the central black hole have always relied on the latest technological advances in observational astronomy. The closer a star is to the central black hole, the stronger the black hole’s influence on its orbit; monitoring the orbits of those stars, at a distance of 27000 light-years, requires observers to distinguish minute details in position, and closely-crowded light-sources.
The considerable advancements in accuracy that allowed for the precise tracking stellar orbits around Sagittarius A* in the early 2000s, for instance, are related directly to the introduction of Adaptive Optics for large telescopes. Adaptive Optics is an active system that compensates for the slight shifts in position caused by atmospheric turbulence – in everyday terms, the twinkling of the stars. But when it came to measuring relativistic effects, even that advanced technology could only get the observers so far. The reason is that the relativistic effects in question are rather small.
Relativistic effects near the black hole SgrA*
Specifically, as a star orbits the black hole, several effects predicted by Einstein’s theory of general relativity come into play. The first effect concerns time: Both due to the star’s high speed and due to its proximity to the black hole, an external observer will see that time on the star passes more slowly. This effect is known as time dilation.
The second effect concerns the shape of the orbit. In classical mechanics, a single object orbiting a mass moves on an elliptical orbit, with the mass in one of the focus points – Kepler’s first law, first formulated in the early 17th century as astronomers struggled to understand planetary motion in our solar system. General relativity predicts a minute change: On an elliptical orbit, there is a point called periapsis, where the orbiting object is closest to the attracting mass. In the classical case, on a purely elliptical orbit, the periapsis has a fixed location.
General relativity predicts what is known as periapsis precession: Over time, the orbital ellipse rotates as a whole, so along each completed orbit, the periapsis will be shifted a little further around the attracting mass. Viewed as a whole, the resulting orbit has the shape of a rosette, with the different nearly-ellipses of each orbit as petals. This effect is known as “Schwarzschild precession”, since the model that describes the gravitational influence of spherical bodies in general relativity is the so-called Schwarzschild solution. A significant part of this effect is due to the curvature of space near the central mass. This effect has been measured within our Solar System, notably for the planet Mercury. It has also been measured for binary stars with at least one neutron star, but never for the motion of a star around a supermassive black hole.
GRAVITY: a precision instrument for tracking stars near the black hole
While astronomical cameras that include adaptive optics have proven their worth over the past 20 years, detecting the Schwarzschild precession for the star S2 called for a considerably higher precision. That is why, in the early 2000s, a group of astronomers led by Frank Eisenhauer (MPE) and including Wolfgang Brandner (MPIA) developed a new type of instrument: GRAVITY, an interferometric instrument in the near-infrared, with its own adaptive optics module, and capable of combining light from all four of the 8-meter-telescopes that make up ESO’s Very Large Telescope. The combination makes for considerably higher resolution: The larger the diameter of a telescope, the finer the details it can discern. When it comes to resolving small details, combining light waves from the VLT unit telescopes with the help of an interferometer is equivalent to observing with a single telescope 130 meter in diameter. In the final design, GRAVITY included its own adaptive optics control system, called CIAO, one at each of the VLT’s 8 m telescopes. CIAO was constructed and built at MPIA, and increases the sensitivity of GRAVITY by a factor between 50 and 100, allowing for the observation of particularly faint objects.
GRAVITY saw first light in January 2016 (MPIA release), and successfully targeted the star S2 in June of that year (MPIA release). The astronomers knew that their main chance would come on May 19, 2018. That was the day when S2 would again have its closest approach to the supermassive black hole, and thus the part of its orbit where relativistic effects are expected to be strongest. While the black hole itself is, of course, invisible, there is always some gas or similar matter in its direct vicinity, which GRAVITY is sensitive enough to detect in the infrared. Between late March and late June 2018, GRAVITY could directly measure the apparent distance between the black hole and S2 with an accuracy of 30 micro-arc seconds – equivalent to tracking the relative position of two candles on the Moon with an accuracy of better than 6 centimeters. With the help of these measurements, plus observations with the NACO camera and the spectrograph SINFONI, the astronomers were able to show that the observations agree with relativistic predictions for the time dilation, which shifts the wavelength of the star’s light by a tiny 0.003%. The agreement between the observations and the predictions on the basis of general relativity is was within 15% (MPIA release).
Measuring Schwarzschild precession with GRAVITY
Now, with additional GRAVITY measurements, the group has detected, for the first time, the Schwarzschild precession in the orbit of the star S2. Thomas Henning, director at the Max Planck Institute for Astronomy, says: “In order to reach this goal the various instruments parts, including the MPIA adaptive optics system, have to work together in a precisely coordinated way with the infrastructure of the Very Large Telescope Interferometer.“ With the new data, the astronomers determined the direction of motion of S2 more than a year before the closest approach and also more than a year after. The directions were fixed by 193 observations with the NACO camera, which has been tracing S2 since 2001, including 75 direct observations of distances between S2 and the black hole taken at times when the black hole was visible to NACO due to infalling matter (“flares”), plus 54 direct measurements using GRAVITY and an additional 92 spectroscopic measurements.
The best fit of the measurements to a relativistic orbit shows a precession effect somewhere between 0.196 and 0.272 degrees per orbit, compared with the relativistic prediction of 0.202 degrees – an agreement between observation and theory within 17%, with the measurements fully consistent with general relativity. "This famous effect — first seen in the orbit of the planet Mercury around the Sun — was the first evidence in favour of General Relativity. One hundred years later we have now detected the same effect in the motion of a star orbiting the compact radio source Sagittarius A* at the centre of the Milky Way” says Reinhard Genzel, Director at the Max Planck Institute for Extraterrestrial Physics (MPE) in Garching, Germany and the architect of the 30-year-long programme that led to this result.
Of astronomical interest is that the agreement also sets a limit on the mass that can be present around the supermassive black hole, within the orbit of the star S2: A compact mass (such as another, smaller black hole) in that region cannot have more mass than 100 solar masses. A diffuse mass, such as a large gas cloud, is unlikely to have a mass of more than about 8000 solar masses, consistent with similar limits derived from more complete studies of stellar motions in the vicinity.
This is the first time orbital relativistic effects of this kind have been measured for a star orbiting a supermassive black hole. The next breakthrough of this caliber will likely need to wait for ESO’s Extremely Large Telescope (ELT) currently under construction in Chile, and slated for “first light” in 2025. With the ELT, and provided there are stars that can be observed close enough to the black hole, astronomers might even be able detect the effect of the black hole’s rotation on space and time. Rotating black holes “drag” the surrounding space, and that effect could be measured using appropriately precise measurements of a nearby star’s orbit.
Background Information
This research was presented in the paper “Detection of the Schwarzschild precession in the orbit of the star S2 near the Galactic centre massive black hole” and is accepted for publication in the journal Astronomy & Astrophysics.
- Research paper (ESO)
- Original article at Astronomy and Astrophysics
- ESO press release
- MPE press release
The MPIA researchers involved are Wolfgang Brandner, Thomas Henning, Stefan Hippler, and Silvia Scheithauer.
The GRAVITY Collaboration team is composed of R. Abuter (ESO), A. Amorim (Universidade de Lisboa - Faculdade de Ciências, Portugal and Centro de Astrofísica e Gravitação, IST, Universidade de Lisboa, Portugal [CENTRA]), M. Bauböck (Max Planck Institute for Extraterrestrial Physics, Garching, Germany [MPE]), J.P. Berger (Univ. Grenoble Alpes, CNRS, Grenoble, France [IPAG] and European Southern Observatory, Garching, Germany [ESO]), H. Bonnet (ESO), W. Brandner (Max Planck Institute for Astronomy, Heidelberg, Germany [MPIA]), V. Cardoso (CENTRA and CERN, Genève, Switzerland), Y. Clénet (Observatoire de Paris, Université PSL, CNRS, Sorbonne Université, Université de Paris, Meudon, France [LESIA], P.T. de Zeeuw (Sterrewacht Leiden, Leiden University, The Netherlands and MPE), J. Dexter (Department of Astrophysical & Planetary Sciences, JILA, Duane Physics Bldg.,University of Colorado, Boulder, USA and MPE), A. Eckart (1st Institute of Physics, University of Cologne, Germany [Cologne] and Max Planck Institute for Radio Astronomy, Bonn, Germany), F. Eisenhauer (MPE), N.M. Förster Schreiber (MPE), P. Garcia (Faculdade de Engenharia, Universidade do Porto, Portugal and CENTRA), F. Gao (MPE), E. Gendron (LESIA), R. Genzel (MPE, Departments of Physics and Astronomy, Le Conte Hall, University of California, Berkeley, USA), S. Gillessen (MPE), M. Habibi (MPE), X. Haubois (European Southern Observatory, Santiago, Chile [ESO Chile]), T. Henning (MPIA), S. Hippler (MPIA), M. Horrobin (Cologne), A. Jiménez-Rosales (MPE), L. Jochum (ESO Chile), L. Jocou (IPAG), A. Kaufer (ESO Chile), P. Kervella (LESIA), S. Lacour (LESIA), V. Lapeyrère (LESIA), J.-B. Le Bouquin (IPAG), P. Léna (LESIA), M. Nowak (Institute of Astronomy, Cambridge, UK and LESIA), T. Ott (MPE), T. Paumard (LESIA), K. Perraut (IPAG), G. Perrin (LESIA), O. Pfuhl (ESO, MPE), G. Rodríguez-Coira (LESIA), J. Shangguan (MPE), S. Scheithauer (MPIA), J. Stadler (MPE), O. Straub (MPE), C. Straubmeier (Cologne), E. Sturm (MPE), L.J. Tacconi (MPE), F. Vincent (LESIA), S. von Fellenberg (MPE), I. Waisberg (Department of Particle Physics & Astrophysics, Weizmann Institute of Science, Israel and MPE), F. Widmann (MPE), E. Wieprecht (MPE), E. Wiezorrek (MPE), J. Woillez (ESO), and S. Yazici (MPE, Cologne).